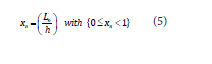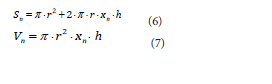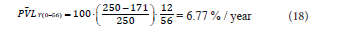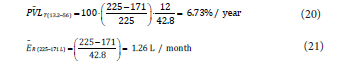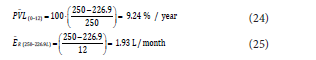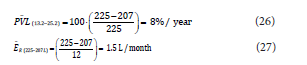Journal of Food Processing & Beverages
Download PDF
Research Article
Optimized Criterion Based on the Surface Area to Volume Ratio for Wood Casks Re-Filling Time Calculation During Long-Term Rum Maturation Process
Del Toro Del Toro N1, Ayan Rial J1, Jan Yperman2*, Vandamme D2, Carleer R2 and Crespo Sariol H3
1 Santiago de Cuba rum factory, Santiago de Cuba, Cuba
2 Analytical and Circular Chemistry, Hasselt University, Agoralaan, building D, 3590 Diepenbeek. Belgium
3 Centre of Neurosciences Signal and Images Processing, Applied Acoustic Research Group, Universidad de Oriente, Santiago de Cuba, Cuba
2 Analytical and Circular Chemistry, Hasselt University, Agoralaan, building D, 3590 Diepenbeek. Belgium
3 Centre of Neurosciences Signal and Images Processing, Applied Acoustic Research Group, Universidad de Oriente, Santiago de Cuba, Cuba
*Address for Correspondence: Jan Yperman, Analytical and Circular Chemistry, Hasselt University, Agoralaan, building D, 3590 Diepenbeek. Belgium E-mail Id: jan.yperman@uhasselt.be
Submission: 16 August 2023
Accepted: 11 September 2023
Published: 15 September 2023
Copyright: © 2023 Del Toro Del Toro N, et al. This is an open-access
article distributed under the Creative Commons Attribution License,
which permits unrestricted use, distribution, and reproduction in any
medium provided the original work is properly cited.
Keywords: Rum; Barrel; Maturation; Surface Area to Volume Ratio;
Optimization; Volume Loss
Abstract
In this work, the behavior of the surface area to volume ratio (s/v)
in five types of standard casks for rum ageing is mathematically
modeled in function of the liquid volume. The (s/v) variation is discussed
and compared between the two barrel storing systems in cellars
(vertical and horizontal). The ratio is applied as a parameter to obtain
an optimized criterion for filling volume and re-filling time of wood
casks during the long-term rum maturation process focused on volume
loss reduction and barrels preservation. An example of re-filling time
calculation and volume loss assessment for rum ageing in a traditional
250 L hogshead barrel is presented and debated.
Introduction
Rum is a distilled beverage obtained from the fermentation of
sugar cane molasses, a sub-product from the sugar cane production.
For the specific case of the rum produced in Cuba, the fresh distilled
spirit (known as “Aguardiente”) is a translucent, shiny, full-bodied
drink with no suspended particles. The production process comprises
the following fundamental stages: (I) Growing of sugar cane, (II)
Obtaining the molasses from sugar cane production process (III)
Molasses fermentation (usually within 24-26 h of fermentation time),
(IV) Distillation (column system in a continuous processis applied),
(V) Natural Ageing/Maturation in white oak (Quercus Alba)
wood during a timed period in order to improve its organoleptic
characteristics and (VI) Mixing [1,2]. In the case of high-quality
products, at least two ageing stages are required. The first corresponds
to the original spirit (Aguardiente). The second corresponds to the
‘base rum’, which consists of a mixture of aged spirits with a distillate
for rum (both filtered through activated carbon) and purified water.
In the specific case of extra aged rums, the use of a certain proportion
of ‘base rum’ that has undergone a third ageing stage is required.
The inclusion of additional ageing stages is optional and is left to
the discretion of the Cuban rum masters. During the ageing stages,
the Cuban rum masters pay special attention to the sensory attributes
of the aged spirit, as it is a decisive element in determining the sensory
profile of the future rum. Among other aspects, they have to select
the characteristics of the barrel (white oak, size and period of time
in use) employed for each of the stages in the ageing process (two as
a minimum) in order to achieve the typical sensory profile required
for each of the stages [1]. Throughout the ageing process, a series of
reactions take place resulting in changes in chemical composition
and organoleptic properties of the rum. These changes will give rise
to modifications in its final quality, due to the complex aroma from
wood. Two variables are fundamental in the ageing process: the
ageing time and the quality of the barrel wood. Barrels are directly
involved in the produced changes on rum’s composition due to the
transfer of oxygen and phenolic and aromatic compounds from wood
to rum [1-11]. Barrels have another important function than those
of serving as a container. An oak barrel acts as an active vessel that
reacts and releases chemical compounds into the rum, improving its
physical, chemical and sensory properties. Depending on its origin,
age, thickness, uses, roasting, size and the contact time, the acquired
properties are different [1,3,12-14]. Wood barrel ageing improves not
only color and mouthfeel, but also increases aroma complexity due to
the extraction of compounds present in the wood. These compounds
include cellulose, hemicellulose, lignin, acids, sugars, terpenes, volatile
phenols and lactones [3,12-14]. Therefore, an ageing period in the
wooden barrel is required to attain sensory fullness and high quality.
Despite its many advantages, natural ageing in barrels has several
drawbacks. Barrels are expensive to produce, have limited lifetime
and generate a significant volume loss due to liquid evaporation [2].
The blends of spirits and the different base rums used in each of the
stages of the ageing process, carried out under the guidance of the
Cuban rum master, are decisive in achieving the finished rum with
proper sensory characteristic balance of taste, color and aromas.
In Cuban rum cooperage technology, used whisky barrels
are generally applied for rum maturation since the barrel wood
exchange/extractable compounds have been already lowered by a
previous contact with whisky. Thus, rum technology is more focused
on potentiating the oxidation ageing mechanisms in long-term aged
products [12-14]. In cooperage technology, the volume loss during
ageing depends basically of the wood permeability, which is defined
as the ability of the wood to allow fluid flow to pass through it without
altering its internal structure. In general, fluid flow through wood
can occur in two ways: (1)As in a porous solid following Darcy’s law
(the bulk flow of fluids through the interconnected voids of the wood
structure under the influence of a static or capillary pressure gradient)
and (2)Due to diffusional mechanisms, also known as permeation.
In turn, diffusion may occur as inter-gas diffusion, which includes
the transfer of water vapor through the air in the wood cell lumen,
and bound water diffusion, which occurs inside the cell walls of the
wood [4]. In the complex case of an oak wood barrel stave during
rum ageing, both phenomena occur simultaneously. The liquid (as
bulk flow) penetrates into the wood, which is a porous solid, due to
the hydrostatic pressure of the liquid, causing the water vapor and
ethanol from the rum inside and the oxygen from the air outside
to diffuse through the wood due to the concentration difference
between the inside and outside of the barrel [15-18]. In this manner,
the evaporation of the liquid (volume loss) through the wood barrel
occurs in two phases: an initial phase or transition state in which
wood is impregnated until a steady state is achieved and a second
phase or steady state in which the impregnation flow entry of fluid
into the wood has the same magnitude as the evaporation outflow to
the exterior [4].
The volume loss is an intrinsic part of the ageing process. As
previously described, the barrel acts as a semi-permeable membrane
that allows evaporation from the cask and migration of air into the
barrel, because of its porous structure [2].
The volume loss during maturation strongly depends on external
factors related with the climatic conditions of the cellar such as
air humidity, velocity and temperature. But also, internal factors
corresponding to the wood and liquid properties (wood porosity/
permeability, density, stave preparation and morphology of the
wood fiber), as well as the alcohol content of the liquid, its density
and viscosity have a significant influence [2,19-21]. The evaporation
loss during the ageing process is an indubitable economic concern for
spirit producers. Therefore, efforts to diminish and/or control the loss
during ageing are crucial in order to optimize the production process
and to reduce the associated costs [2].
Apart from the external and internal factors previously mentioned
that influence evaporation during spirit ageing, one of the most
important aspects is the barrel size. The percent volume evaporated
from the barrel increases as a direct function of the cask surface area
to volume ratio (s/v) expressed in m2 of wood surface per m3 of liquid
volume in the barrel [19,21].
Production economy favors the larger size barrels due to the
large storage capacity per surface of the wood and less evaporation
rate during ageing, whereas ease of movement and earlier maturation
are favorited by a smaller size [22-25]. A compromise between these
opposite aspects has led to the adoption of barrels with a capacity
ranging between 200 and 500 L in spirits such as whisky and rum.
The ratio of (s/v)most used traditional barrels of 200-250 L is roughly
calculated at (s/v)= 9 m-1 which is equivalent to 90 cm2 /L [26]. Due to its significant (s/v) importance for alcoholic beverages technology,
the effect of ratio during the ageing process has been widely debated
in several publications [22-26]. In that sense, experiments have been
conducted in order to study the influence of different barrel sizes
during the ageing process of spirits and wine, focused mainly on the
chemical/sensory profile and evaporation loss [2,22-26].
However, in the case of spirits with long ageing periods (more
than 3 years of maturation) as the volume of the liquid in the barrel
decreases due to the evaporation, the wetted surface area by the liquid
into the barrel also changes. Therefore, opposite to the traditional
consideration that the (s/v) ratio in the barrel is a constant value, this
ratio actually changes in function of the ageing time.
Following this approach, the (s/v) ratio of any barrel will be
ranging from a constant value (barrel completely filled) which
certainly depends on the cask size and a final value that will tend to
infinity when the volume of the liquid contained in the barrel tends to
zero. This will be mathematically demonstrated further on.
Based on that, it can be the case that two barrels of different sizes
might have equal (s/v) ratio at certain volume (filling level) of liquid.
In addition, as extensively reported [2,14-26], the change of (s/v)
ratio in a wood barrel influences not only the wood-liquid chemical interaction and ageing reaction kinetics but also the volume loss. The higher the (s/v) ratio (like in small barrels), the higher is the percent of
volume loss and the faster the ageing process [2, 12-18, 22-26]
So far, no references were found concerning to the discussion
of the change of (s/v) ratio in function of the liquid level in a barrel
during the ageing process. Specifically, for the long-term spirits
maturation (and especially in tropical countries) the barrels suffer a
significant reduction of its liquid volume due to evaporation with an
average volume loss ranging from 6-13% per year depending on the
climatic conditions in non-conditioned cellars [2].
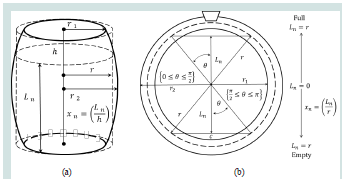
In cellars, barrels can be stored for spirits maturation in
vertical or horizontal position as presented in [Figure 1a,b]
respectively. In order to increase the storing/logistic efficiency of the
cellar’s space, spirit producers have introduced the palletized system
of ageing which consist on storing the barrels in vertical position and
supported by pallets.
Palletized strategy has advantages compared with the traditional
racked ageing system (barrels horizontally stored). The vertical
arrangement not only increases the volume storage yield (L/m2) up to
20% but also improves the work dynamics in the cellar, which in turn
impact significantly on the logistic/production capacity of the spirit
manufacturing. However, after many years of using the traditional
rack system of ageing, volume loss is still under assessment for
rum ageing in palletized system. The barrel geometry influences
the production processes, the cost of the barrel and its storage and
consequently, the added value of the rum [27].
Several mathematical methodologies have been applied to
geometrically describe the wood barrels used in wine and spirit
industry. Until today, tradition has passed the use of empirical and
simplified formulas that relate the barrel volume to the following
shape parameters: belly diameter, head hoop and length or even the
distance from the bunghole to the head/bottom corner. Ten empirical
and simplified formulas have been reported for barrel volume
calculation [27].
Although the error of employing these formulas fluctuates in
the range of 4-10% between them [27], its main advantage is their
simplicity which is a very suitable aspect for practical purposes in
cooperage and cellar management. Additionally, very dedicated and
more complex methods to describe barrel geometric parameters
such as 3D modelling, finite differences method and evolution of
a circumference arc through the infinitesimal calculus have been
reported [27]. Both, empirical and complex models are used for
the economic optimization of the barrel, connected with the (s/v)
ratio. On the other hand, as the barrel continuously loses liquid due
to evaporation, along the time, the upper staves eventually are not
wetted by the liquid. So, in time, if the volume liquid is low enough,
the head space barrel wood dries out, the stave joint separation
increases and the cask becomes less hermetic. At this point, the barrel
suffers a progressive deterioration of its mechanical integrity, ageing
efficiency and in the worst scenario, an irreversible damage.
Therefore, this problem can be avoided if the barrel is re-filled
with liquid of the same maturation time to reach again an adequate
liquid volume. The cask re-filling process is a common managing
strategy in cellars during long-term rum ageing not only to protect
the cask from deterioration but also to optimize the cellar storage
efficiency.
In Cuban rum technology, at a certain volume of liquid, the
content of partially filled casks with the same aged product is used
to complete the cask volume at the original level, thus emptied ones
can be efficiently recovered back to the process cycle. Based on that,
for spirits manufacturers which manage very aged products under
natural ageing technology, it is a continuous concern to find the
optimal re-filling time of the casks.
In general, the re-filling time is in general empirically determined
according to the experience of the specialists and based on the specific
conditions of the cellar. In this work, the behavior of the (s/v) ratio
in function of the liquid volume in five types of standard casks for
rum ageing is mathematically modeled. The change of (s/v) ratio with
the liquid volume is discussed and compared between the two barrel
storing systems in cellars (vertical and horizontal). The (s/v) ratio
is applied as parameter to obtain an optimized criterion for filling
volume and re-filling time of wood casks during the long-term rum
maturation process focused on volume loss reduction and wood
barrels preservation. The presented study might be useful not only
for rum producers but for other specialists and researchers in the
production of aged spirits.
Materials and methods
Mathematic Approach:
The cask geometric shape is a truncated prolate spheroid with
circles of equal radius in top and bottom (head size). The end radius,
commonly defined as the “Head / Top radius” is presented in Figures
1 (a) and (b) as r1. On the other hand, the “belly radius / bilge size”
r2 is the radius of the widest circumference formed by the spheroid
[28]. The total volume and surface area of a cask can be determined
by Eqs. (1) and (2) respectively assuming it has an equivalent cylinder
of radius (r) of the truncated prolate spheroid (this simplified
mathematical approach has been reported as one of the more accurate
models with about 5% of error) [27].
thusThe (s/v)0 ratio corresponds to a fix value which is indeed
characteristic for each barrel depending on its size and geometric
proportions.
When the barrel is full of liquid, the internal wall of the cask is
completely wetted. When the liquid evaporates during ageing, in a
discrete level point “n”, the liquid volume as well as the wetted surface
in the cask will vary in different orders, so the initial (s/v)0 will change
to another (s/v)n value in function of the liquid level/volume.
Vertical position:
Analyzing the simpler case of vertical barrel position[Figure 1a]
and considering the barrel’s geometry as an equivalent cylinder of
radius (r), the liquid volume variation is a linear function of the liquid
level (Ln). Then, any liquid level (liquid volume) in the barrel at a discrete
point “n” can be expressed as a fraction (xn) of the total barrel height
asHowever, when the barrel loses liquid (it is not full anymore) the
upper head is not wetted in vertical position, therefore ( n 0 S ≠ S ) and
equations (1) and (2) can be transformed as an xn function in order
to calculate the wetted surface and the volume of liquid in a discrete
level point “n” as
Sn: Wetted internal surface area of the barrel at a level point “n”
(in m2)
Vn: Liquid volume contained in the barrel at a level point “n” (in m3)
xn: Fraction of liquid level at a discrete point “n”(dimensionless)
Ln: Liquid level in the barrel at a discrete point “n”(in m)
Consequently, by combining Eqs. (5), (6) and (7) gives for vertical position
xn: Fraction of liquid level at a discrete point “n”(dimensionless)
Ln: Liquid level in the barrel at a discrete point “n”(in m)
Consequently, by combining Eqs. (5), (6) and (7) gives for vertical position
(s/v)n: Surface area to volume ratio at a specific liquid level/volume
discrete point “n” (in m-1)
From Eq. (8) it can be defined that lim n xn (SV)n → = ∞ therefore it
is confirmed that the (s/v)n ratio increases as an inverse function of
the liquid level into the cask.
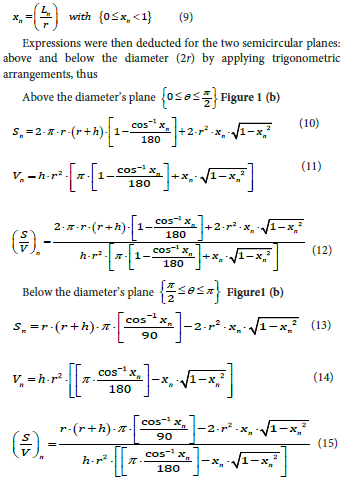
Horizontal position:
The mathematical modeling of the (s/v)n in function of the fraction
of liquid level in the horizontal position of the cask (Figure1 (b)) is
somewhat more complex to obtain. In the circumferential geometry,
the fraction of liquid level is not a linear function of the liquid volume
as presented in the vertical position.
In this caseBarrels:
Five types of whisky barrels with capacities ranging from 40-
120 UK Gallons (182-545 L), typically used in rum industry, were
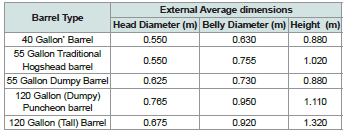
evaluated. [Table 1] presents the external average dimensions of the
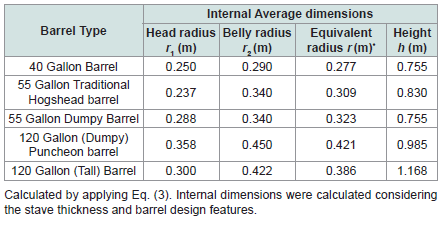
assessed casks. On the other hand, [Table 2] depicts the internal average
dimension calculated by considering the staves thickness (ranging
from 25-32 mm) and barrel design features.Results and Discussion
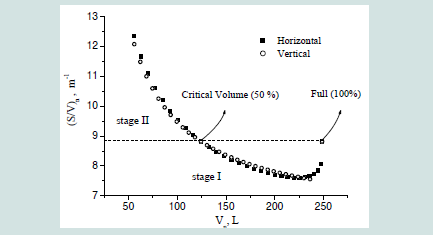
[Figure 2] presents a comparative plotting of the (s/v)n ratio in
function of the liquid volume at the two different storage positions of
the barrel: horizontal and vertical from 250 (full) to 25 L. Practically
the same behavior of (s/v)n ratio was observed with minor differences
between vertical (using Eqs. (4-8)) and horizontal positions (using
Eqs. (9-15)) of the assessed traditional hogshead whisky barrel. All
the studied barrels [Table 1 and Table 2] presented the same pattern
without almost any difference between both storage positions.
Therefore, it can be concluded that the barrel position has not a
significant influence on the surface area to volume ratio variation in
function of the liquid volume.
Based on the (s/v)n ratio, it is possible to identify two stages of the
barrel during ageing: (I) First half period and (II) Second half period.
Since the objective of this work is basically focused on diminishing
the volume loss and cask deterioration during ageing, it is highly
advisable to keep the barrels in stage (I) thus avoiding as much as
possible the stage (II).
Therefore, the optimized criterion for filling volume and re-filling time of wood casks will be restricted to the stage (I) range analysis.
Therefore, the optimized criterion for filling volume and re-filling time of wood casks will be restricted to the stage (I) range analysis.
Figure 1: Diagram of the general geometrical parameters of wood casks from
different positions: vertical (a) and horizontal (b). xn: Fraction of liquid level.
Ln: Liquid level (in m) and θ: filling angle (degrees). Dash line represents the
equivalent cylinder of radius (r).
When the hogshead barrel is full (100% filled), the calculated
(s/v)0 ratio was 8.882 m-1 ≈ 90 cm2/L [Table 3] which is consistent with the reported value [11]. However, when the liquid volume in
the hogshead barrel decreases (let’s say, due to evaporation losses),
the (s/v) ratio diminishes under this initial value (dash line). Based
on [Figure 2], when the hogshead barrel is at half capacity (50%), the
(s/v)n ratio is equal to the initial value as the volume and surface area
are both proportionally reduced to 50%.
On the other hand, if the volume of liquid decreases below the 50%
of the total capacity of the barrel, the (s/v)n ratio abruptly increases
following an exponential trend which (as previously discussed) reach
an infinite value when Vn = 0. Since the volume loss during ageing is
a direct function of the (s/v)n ratio, it is expected that below the 50%
of the hogshead barrel capacity the evaporation rate will increase also
following the same tendency. Therefore, it can be stated that at 50%
of capacity, the barrel reaches a critical volume of liquid, a sort of an
inflection point. Under that liquid volume, the evaporation losses will
be significantly higher also leading to a fast deterioration of the barrel
mechanical integrity due to the wood stave’s desiccation process.
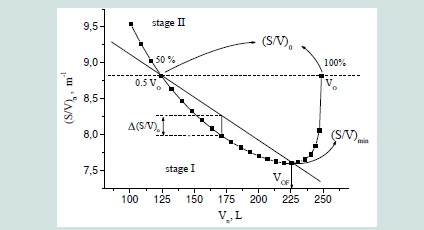
[Figure 3] shows a magnification of [Figure 2] focused on the stage (I)
range of the hogshead barrel. The stage (I) comprises also interesting
aspects to take into account for the optimization analysis. However, at
the beginning, even when the liquid volume decreases just a few liters,
the (s/v) value abruptly diminishes to reach a minimum (optimal)
value. In the hogshead barrel (250 L), the minimal (s/v)min value is
equal 7.624 m-1 and this value is reached when the liquid volume in
the barrel is VOF= 225 L (see table 3).
With 10% less of the total barrel capacity, the (s/v)n ratio decreases
approximately 14 %. After this minimal point, the (s/v)n ratio increases
as inverse function of the liquid volume but with a significant less
abrupt slope compared with the (s/v)n ratio change found from 250 to 225L.
Regarding the impact of the (s/v)n ratio on the volume loss, at the
optimal value of (s/v)min, the barrel will suffer from minimal volume
loss due to diffusional evaporation. Therefore, each barrel has an
optimal operational capacity or optimal filling volume (VOF) in which
the diffusional evaporation loss finds a minimum. The optimal filling
volume was calculated for the different whisky barrels assessed (Table
2) and values are presented in [Table 3].
Figure 2: Comparative plotting of the (s/v) behavior in function of the liquid
volume (range: 55-250 L) at the two different storage positions (horizontal(Eqs.
(9-15) and vertical (Eqs. (4-8)) in a 250 L traditional hogshead barrel.
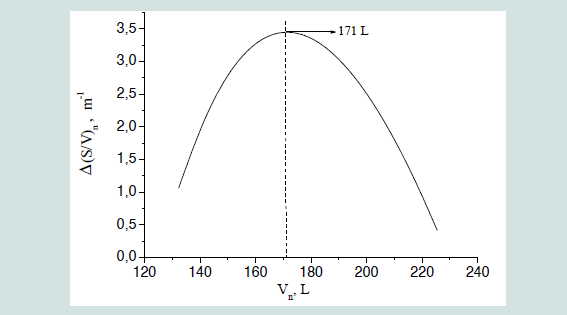
Figure 3: (s/v) ratio in function of the liquid volume in stage (I) for a traditional
(250 L) hogshead whisky barrel. Magnification of the stage (I) from Figure 2.
VOF: Optimal filling volume (in L). Δ(S/V)n: Differences of surface to volume
ratio at each Vn point between the curve and the line applied as optimization
function.
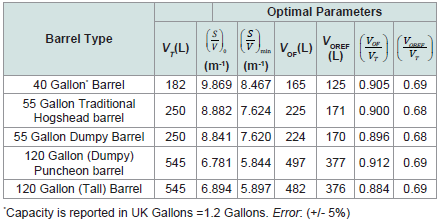
Table 3: Optimal parameters for filling and re-filling volumes determined for
different types of whisky barrels used for rum production.
From this result, a conclusion can be derived: instead of filling it
to 100%, it is better to fill the barrel till its optimal volume (VOF) to be
stored in the cellar for spirit ageing in order to reduce the diffusional
evaporation losses. This last statement and its demonstration will be
discussed later on.
Filling a barrel below its full capacity could seem to be a
contradictory idea, because it reduces the space yield efficiency used
in the cellar. However, according to the experience of traditional
spirit producers, a small air space is empirically left during cask
filling in cellars to counteract the pressure changes due to the liquid
expansion and contraction in function of the temperature fluctuation
and also potentiate the gas-liquid contact interface for oxidation
reactions during maturation which in turn improves the organoleptic
attributes of the rum. Nevertheless, apart of the applied empirical
approaches which are very specific for each producer, the effect of
the barrel filling volume and the re-filling time on the volume loss
during spirits maturation has not been clearly elucidated so far and
the subject is still under discussion among spirit producers
From Figure 3, it is noticeable that the evaporation loss from V0 to
V0F occurs in a faster way compared with the liquid volume reduction
from V0F to 0.5 V0 which is in line with the equilibrium concept.
Additionally, rum producers in Cuba locally report a relative
increment of the evaporation loss for short-term (less than 15
months) aged products which it is in line with the (s/v)n vs Vn pattern
observed in [Figure 3].
The barrel will tend to reach the minimum as it is not in the
optimal point when it is full. As it will mathematically modelled and
discussed later, the evaporation loss rate in the range of V0 - V0F (250-
225 L) volume change period is significantly higher than for V0F to 0.5
V0 (225-125 L) period. Thus, the analysis has to be conducted to see
if it is beneficial to fill the barrel fully since the liquid will be rapidly
evaporated and is lost anyway.
Another objective in this work is the optimal refilling time of the
barrel during maturation. From Figure 3, it can be deducted that the
refilling volume for a barrel is located in the region of V0F to 0.5 V0
(225-125 L in the case of the hogshead barrel). The optimal volume
for barrel refilling (VOREF ) can thus be mathematically determined.
Drawing a line between coordinates points [(s/v)min; v of] (Figure 3) and calculating the difference at each volume Vn in the barrel between the curve and the line as
the optimization function, the inflection point in the curve trajectory
indicates the optimal refilling volume which will be observed at the
maximal difference Δ (s/v)max [Figure 4].
In this case, for the traditional hogshead barrel, the optimal
refilling volume (VOREF) was found at VOREF = 171 L (+/- 8.6 L). This indicates that when the barrel reaches this volume, it is the moment to re-fill it in order to keep the evaporation loss controlled and preserve the barrel’s mechanical integrity.
In Table 3, a summary of the optimal parameters for casks filling and re-filling volume determined for different types of whisky barrels used for rum production are presented. As reported in [19-21], the smaller the barrel size, the higher is the (s/v)0 value. The shape of the barrel (dumpy or tall) also modifies the (s/v) value. For dumpy shape (more like a square cylinder) the calculated (s/v)0 value is slightly
lower than for the all-shaped designs. Furthermore, the same applies for the (s/v)min parameter.
An important aspect to notice from [Table 3] is that independently
of the found differences between optimal parameters of the barrels
(which in turn depends on the relative barrel dimensions) it can be
assumed for practical purposes that the minimal value of (s/v)n ratio
for a typical barrel is when the cask is about 90% filled (+/- 5% of
error based on the accuracy of the simplified formula to calculate r
(Eq.(3) and the optimal volume for re-filling is when the barrel losses
are around 30% of its total capacity.When the optimal re-filling
volume of a barrel is determined and the evaporation loss percent in
the cellar has been established by experimental/empirical studies, the
re-filling time can be then defined.
In order to clarify the method, an example of a numerical
calculation approach to determine the optimal re-filling time is
presented. Calculation results are presented in [Table 4] under the
following conditions:
a) Barrel type: (55-Gal Hogshead whisky barrel/ 250 L)
b) Product: ‘base rum’ (52-55 % v/v)
b) Maximal percent of evaporation loss at full barrel: 11 %/ year
(Caribbean region)
c) Temporal discrete step for the refilling time calculation:
Δt = 3 months (1/4 of year)
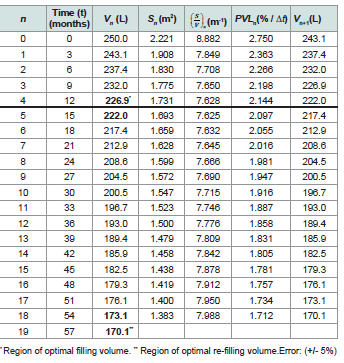
Similar to the numerical Euler’s method, the first row (n = 0) in
Table 4 is completed with the initial values calculated at full barrel
conditions V0 = 250 L. The initial partial volume loss PVLn in 3
months (t = n . Δt) is then:
PVLn =11/4= 2.75 % of the volume loss / 3 months
Since the diffusional evaporation is a linear function of the contact
surface area [28, 29], the partial volume loss after a Δt of time PVLn for
(n> 0) is calculated by Eq. (16).
Table 4: Example of a numerical calculation approach to determine the re-filling
time in a 55-Gal hogshead whisky barrel at specific conditions.
to evaporation taking the whole 56 months period as reference can
be calculated as
As presented above, a 11% / year of volume loss was considered at
full barrel. However, the average value after 56 months was about a 40
% lower. This is in line with internal statistics and empiric criteria of
Cuban rum producers about the influence of the ageing time in yearly
volume loss percent.
Also, the average evaporation rate (ER(t)) in the whole period
can be determined as
On the contrary, if the barrel was filled initially to its optimal filling
volume of 225 L (corresponding to 13.2 months by interpolation in
Table 4), the refilling time is then (56 - 13.2 = 42.8 months) around 3
years and 7 months.
Thus, from the optimal filling volume, the average of percent of
total volume loss per year and average evaporation rate in this period
can be calculated as
Comparing both full and optimal initial filling volume, in a
long-term ageing process the average loss is reduced in around
0.15 L/month-barrel which represents just 0.6% less than the yearly
evaporation loss at initial full barrel. In conclusion, for long-term
maturation, the effect of the initial barrel filling volume on the
evaporation loss reduction is not that significant.
However, as it will be demonstrated in the following calculations,
short-term evaporation loss during the spirit ageing process is highly
affected by the initial filling volume of the barrel.
Firstly, if the initial period from full barrel to reach the optimal
filling volume of 225 L is analyzed, it is possible to find significant
differences. As previously mentioned, by interpolation, the time to
reach the 225 L from hogshead full barrel is 13.2 months and the
averaged percent of volume loss per year and evaporation rate in this
ageing period are respectively
In this first period of approximately one year, the average percent
of volume loss per year and the evaporation rate are about 35 % higher
compared with the whole period of 56 months, thus this is consistent
with local reports of Cuban rum producers.
The results confirm the influence of the first ageing period on the
increment of the total volume loss in cellars. At this point, making
an analysis for the short-term ageing process to compare the volume
loss reduction if the barrel is filled at optimal volume against the full
barrel filling.
Let’s consider a 12 months aged product (n = 4), if the barrel
was initially full, the average volume loss and evaporation rate in this
period can be calculated as
In a short ageing period, by initially filling the barrel at optimal
volume, the percent of evaporation loss can be reduced approximately
87% compared with the percent of volume loss at full barrel filling
in the same ageing period (12 months in this case). That represents
a saved volume of around 5.2 L/year-barrel (hogshead barrel). Thus
for 100 barrels in the cellar, 520 L of product can be saved from
evaporation per year. The same calculation method can be conducted
for other barrels types and cellar specific conditions.
Beyond the theoretical results, the proposed method needs to be
adapted for practical purposes and then efficiently applied in different
scenarios of spirit industry according to the specific features of each
producer such as cellar environmental conditions, ageing technology,
cask management strategy among others. Indeed, other math models
can be explored and compared with found results in order to increase
the calculation accuracy for different types of barrels.
Conclusions
The (s/v)n ratio of a barrel during ageing constantly changes in
function of the volume of liquid and therefore the ageing time. This
parameter will range from a constant value (barrel completely filled),
which certainly depends on the cask size, to a final value that will
tend to infinity when the volume of the liquid contained in the barrel
approaches to zero.
Found results demonstrated that for traditional barrels within a
capacity range of 182-545 L:
1) the (s/v)min ratio is practically independent of the barrel storage
position (horizontal or vertical).
2) the (s/v)min ratio and therefore its minimal diffusional
evaporation loss is established when the barrel is around 90% of its
total capacity during short-term (less than 15 months) maturation
process.
3) instead of filling the barrel to 100%, it is better to fill the barrel
till its optimal volume (VOF) to be stored in the cellar for spirit ageing
in order to reduce the diffusional evaporation losses.
4) in order to avoid cask deterioration during long-term spirit
maturation, the barrels have to be re-filled when the liquid volume
reaches 70% of the total barrel capacity.
5) for long-term (more than 3 years) ageing process, the initial
filling volume of the barrel does not have a significant influence on
the average evaporation loss reduction.
6) in a short ageing period, by initially filling the barrel at optimal
volume, the percent of evaporation loss can be reduced approximately
87% compared with the percent of volume loss at full barrel filling in
the same ageing period.
The presented study might be useful not only for rum producers
but for other specialists and researchers in the production of aged
spirits. The proposed method needs to be adapted for practical
purposes and then efficiently applied in different scenarios of
spirit industry according to the specific features of each producer
such as cellar environmental conditions, ageing technology, cask
management strategy among others.
Author Contributions
N. del Toro del Toro and H. Crespo Sariol: conceptualisation,
formal analysis, writing - original draft, validation; N. del Toro del
Toro and J. AyánRial:investigation, methodology, visualisation; H.
Crespo Sariol, J. Yperman, D. Vandamme: project administration,
resources, supervisionJ. Yperman, D. Vandamme, R. Carleer and H.
Crespo Sariol: analysed the calculation methods and math models,
writing - review and editing.
Acknowledgments
The authors would like to thanks the VLIR-UOS project between
Belgium and Cuba for providing funding and granting the support of
the current and future studies.
Conflicts of Interest: The authors declare no conflict of interest.
Compliance with ethical standards: The authors declare that the submitted work is original and is not published elsewhere in any form or language, following the rules of good scientific practice and maintaining the integrity of the research and its presentation.
Conflicts of Interest: The authors declare no conflict of interest.
Compliance with ethical standards: The authors declare that the submitted work is original and is not published elsewhere in any form or language, following the rules of good scientific practice and maintaining the integrity of the research and its presentation.